The majority of limit-cycle oscillators have the intrinsic property of amplitude dependent frequency or shear, meaning that transient trajectories corresponding to different amplitudes have different average frequencies. This property does not typically affect the stability of a solitary oscillator and is best illustrated by invariant sets called isochrons [1]. Substantial shear is intrinsic to semiconductor lasers, which are widely applied examples of nonlinear oscillators described by a complex-valued electric field, \(E(t)\), and a real-valued population inversion, \(N(t)\). Its physical origin is the dependence of the laser-resonator frequency on population inversion, which is quantified by the single parameter \(\alpha\). In our paper [2] we demonstrate how this seemingly innocent property can lead to the rich variety of instabilities and chaos displayed by externally perturbed semiconductor lasers.
We were guided by the work of Wang and Young [3] who proved that any hyperbolic limit cycle, when suitably perturbed, can be turned into observable chaos (a strange attractor). This result is obtained for periodic and discrete-time kicks that deform the otherwise stable limit cycle. A key concept is the creation of Smale horseshoes via a stretch-and-fold action due to an interplay between the kicks and properties of the phase space flow. In systems without shear the stretch-and-fold action requires very carefully chosen kicks that need to be in both the radial and angular directions. In contrast, in the presence of shear, it may be sufficient to kick in the radial direction alone and let the natural forces of shear provide the stretch-and-fold action.
These effects are illustrated in the animation. The stable limit cycle of a solitary laser with two complex conjugate Floquet multipliers is shown in red and henceforth referred to \(\Gamma\). Because the limit cycle is rotationally symmetric, motion along \(\Gamma\) can be frozen in a suitable reference frame so as to not obscure any stretch-and-fold action. Kicks modify the electric field amplitude, \(|E|\), by a factor of \(0.8*sin(4*\arg(E))\) at times \(t = 0\), \(0.25\), \(0.5\), and \(0.75\) but leave its argument, \(\arg(E)\), and population inversion, \(N\), unchanged. For \(\alpha = 0\), kicks leave each point on its original isochron which is given by a constant phase, \(\arg(E) = const\). Therefore, all points on the black curve rotate with the same frequency about the origin of the \(E\)-plane and no folds appear as the black curve settles back to \(\Gamma\). However, for \(\alpha = 2\), kicks move most points to different isochrons which are now logarithmic spirals given by \(\arg(E) + \alpha*\ln(|E|) = const\). As the black curve converges to \(\Gamma\), points with larger amplitudes \(|E(t)|\) rotate faster on average. This gives rise to an intricate stretch-and-fold action that is additionally enhanced by the spiralling transient motion about \(\Gamma\). Folds and horseshoes are formed even though the kicks are applied in the radial direction alone.
Although laser systems usually have continuous-time perturbations that may not be periodic, the rigorous results in conjunction with numerical computations give new valuable insight as to why vast parameter regions of persistent chaos appear in externally perturbed lasers with $\alpha$ sufficiently large. The results also suggest that creating observable chaos for \(\alpha=0\) may be difficult, but not impossible.
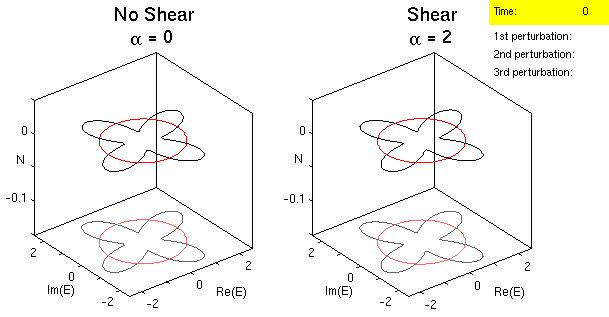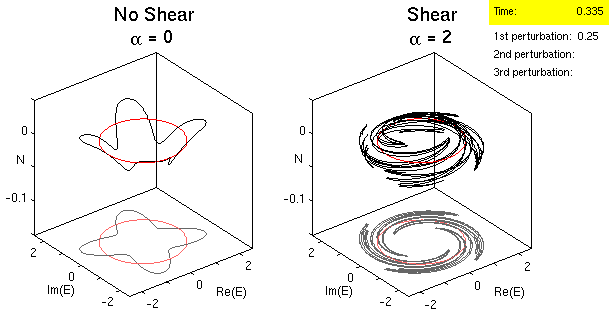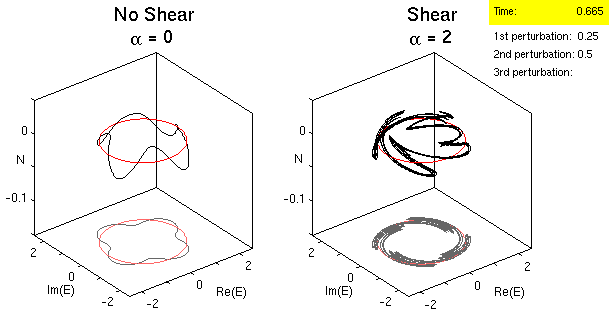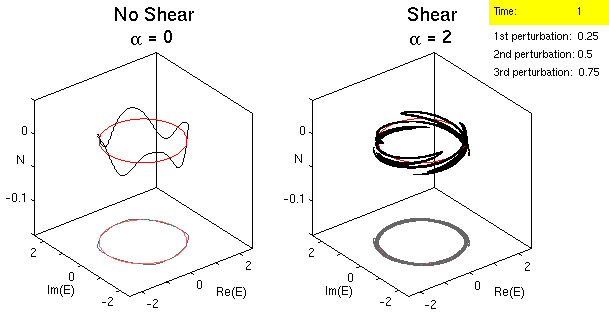
Shear-induced Chaos in Lasers : Animation.
[1]
J. Guckenheimer, "Isochrons and phaseless sets", J. Math. Biol. 3 259-273 (1974/75)
[2] N. Blackbeard, H. Erzgräber and S. Wieczorek, "Shear-induced bifurcations and chaos in coupled-laser models", SIADS 10(2) 469-509 (2011)
[3] Q. Wang and L.-S. Young, "Strange attractors
| Author Institutional Affiliation | College of Engineering, Mathematics, and Physical Sciences Harrison Building University of Exeter |
| Author Email | |
| Author Postal Mail | Harrison Building Streatham Campus University of Exeter North Park Road Exeter EX4 4QF Tel: +44 (0)1392 723628 |
| Notes | this paper appeared in SIADS 10(2): 469-509, 2011. |
| Keywords | coupled lasers, bifurcation analysis, Lyapunov exponents, codimension three, Belyakov bifurcation |