The picture is a space-time plot with time going downward of a chemical concentration in a homogeneous one-dimensional medium. The arising pattern has been called a 'one-dimensional spiral': a self-organized source (in the homogeneous medium!) sends out pulses to left and right in an alternating fashion.
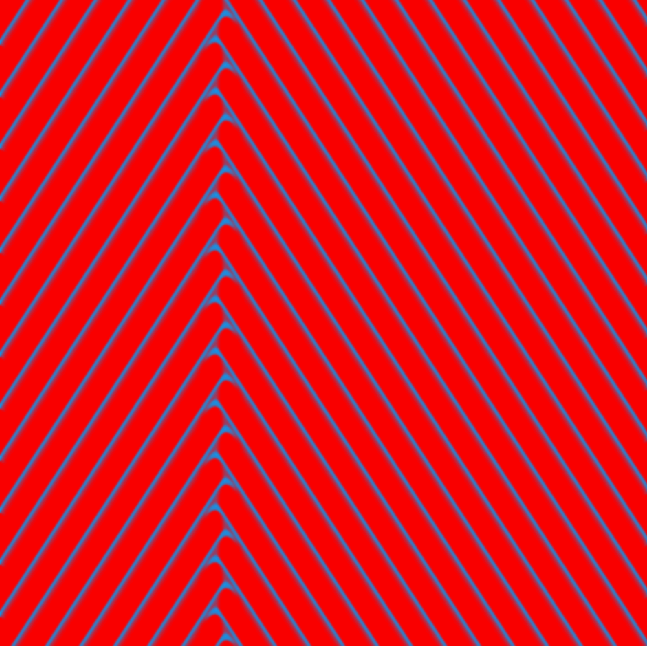
Parameters: \(a=0.84, b=0.15, 1/\epsilon=10.8\), domain length=400, Neumann boundary conditions. Numerics is first order finite differences with explicit Euler in time.
The underlying equation is the two-component reaction-diffusion system
\begin{eqnarray}
u_t &=& \frac 1 \epsilon u (u-1) \left(u- \frac{b+v}a\right) + u_{xx}\
v_t &=& f(u) -v
\end{eqnarray}
where
$$f(u) = \cases{0,\quad0 \leq u < 1/3,
1-6.75 u (u-1)^2, \quad1/3 \leq u \leq 1,
1,\quad 1 < u}$$
which has been derived to model aspects of CO-oxidation on a platinum surface, see [M. Bär et al, J. Chem. Phys. 100 (1994) 1202]. In the picture, red corresponds to low and blue to high concentration of the v-component.
| Author Institutional Affiliation | Centre for Mathematics and Computer Science (CWI), Dept. Modelling Analysis and Simulation (MAS) |
| Author Email | |
| Author Postal Mail | Kruislaan 413, 1098 SJ Amsterdam, the Netherlands |
| Notes | This simulation result has not been published. Similar results can be found in the work of M. Bär et al in the 90s. |
| Keywords | reaction-diffusion system, one-dimensional spiral, self-replication |