Dynamical systems with more than one attracting solution have the problem that the long-time behaviour to the system depends critically on the initial data. This issue is exasperated when a large number of attractors are present. It is interesting to study the ultimate case that infinitely many attractors coexist.
For smooth maps, infinitely many attracting periodic solutions can coexist near homoclinic tangencies (this is part of Newhouse's phenomenon), but this is difficult to observe numerically. Piecewise-linear maps, on the other hand, do not exhibit homoclinic tangencies in the same fashion, but periodic solutions of such maps are easy to find because one only has to solve linear matrix equations.
The following piecewise-linear continuous map has an infinite sequence of attracting periodic solutions:
$$
\left[ \begin{array}{c} x_{i+1} \\ y_{i+1} \end{array} \right] =
\left\{ \begin{array}{lc}
\left[ \begin{array}{c} -\frac{55}{117} x_i + y_i + 1 \\ -\frac{4}{9} x_i \end{array} \right] \;,
& x_i \le 0 \\
\left[ \begin{array}{c} -\frac{5}{2} x_i + y_i + 1 \\ -\frac{3}{2} x_i \end{array} \right] \;,
& x_i \ge 0
\end{array} \right.
$$
The first figure below shows the basins of attraction of the first eight solutions in this sequence. Different colours indicate different basins and the vertical line is the \(y\)-axis. There is an underlying period-three solution of saddle-type. Its stable and unstable manifolds are coincident and form an invariant quadrilateral (with the period-three solution). The second figure shows basins of the same map but with different values for the four \(x_i\)-coefficients.
Piecewise-linear continuous maps are important because they describe dynamics near border-collision bifurcations of nonsmooth systems. The figures represent instances of a codimension-three phenomenon for which much is known about the periodic solutions, but the fractal structure of the basins has not been explored.
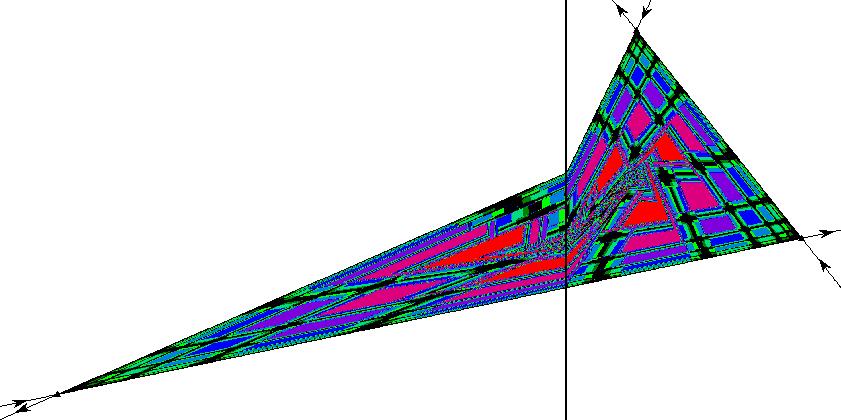
Basins of attraction for the above piecewise-linear continuous map.
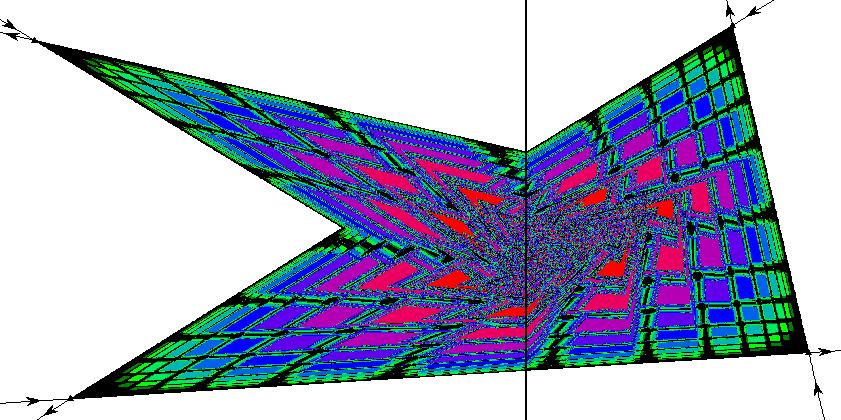
Basins of attraction using different \(x_i\)-coefficients. Here the underlying saddle-type periodic solution is of period four.
\(\bullet\)
D.J.W. Simpson.
Sequences of Periodic Solutions and Infinitely Many Coexisting Attractors in the Border-Collision Normal Form.
Submitted to: Int. J. Bifurcation Chaos.
| Author Institutional Affiliation | Institute of Fundamental Sciences, Massey University |
| Author Email | |
| Author Postal Mail | Institute of Fundamental Sciences, Massey University, Palmerston North, 4442, New Zealand |
| Keywords | basin of attraction, homoclinic tangency, piecewise-linear, border-collision bifurcation |