Alan Turing's chemical theory of pattern formation [5] has given rise to a wide range of fascinating analytical and numerical studies of pattern formation, many of which make use of (and have motivated the development of) tools from nonlinear dynamics. A particular idealization made in Turing's original model is that the spatial domain is fixed, while real development occurs in a complex medium that is, in some sense, always evolving in time.
Growing domains have been shown to give rise to a robust period-doubling bifurcation of spike splitting [1], plausibly being one resolution of the 'robustness problem' of how Turing-type systems, and their associated patterning, can be sensitive to initial data. This period-doubling behaviour has been shown to apply to quite a wide class of systems that grow uniformly in space [6]. Unsurprisingly, non-uniform growth can give rise to more exotic insertion of pattern elements [2], such as in Fig. 1.

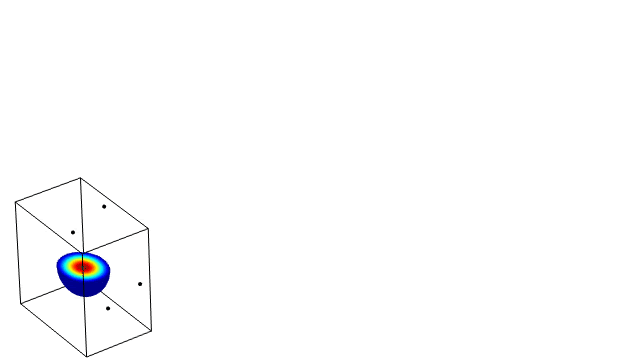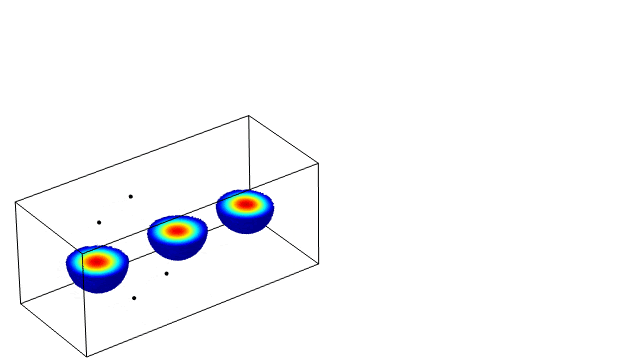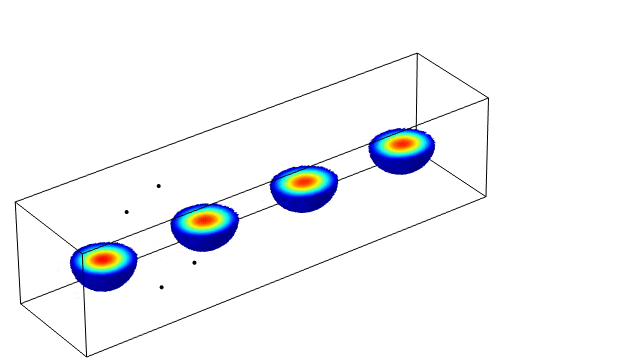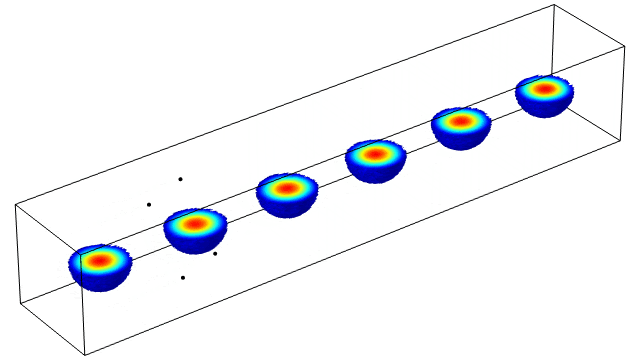
Figure 1: Non-uniform growth can lead to more complex insertion of pattern elements, as shown here in 2D and 3D simulations of Gierer-Meinhardt systems on growing domains. In both simulations, the black dots represent a change in the local growth rate, with the region to the left of these black dots growing more slowly than the region on the right.
One can develop a theory of linear instability for such time-evolving systems when the domain evolution is both prescribed and is of a coordinate-dilational nature [7]. However, linear stability analysis is not especially informative for these systems, especially under regimes where the domain evolution is fast, or in the case of concentration-dependent evolution, wherein `far-from-equilibrium' structures in the solutions can lead to quite exotic domain evolution and subsequent pattern bifurcation. In Fig. 2, we give an example of local domain expansion and contraction that is dependent on the local chemical concentrations. These simulations all have identical setup1 except for a single parameter, representing the rate of local domain evolution, which increases in subsequent plots. For `slow' domain evolution rates, one observes the aforementioned spike-doubling bifurcations. In contrast, for larger values of this parameter, the system exhibits interesting transients, which can include metastable switching between one and two spike solutions, purely oscillatory growth and contraction, steady state profiles with no further net domain growth, and even domain contraction. See [4] for details of the model, including open-source code for exploring it numerically. In contrast to the linear stability theory presented in [7] or the weakly nonlinear analysis of spike-doubling in [6], the proposed model of concentration-dependent growth is autonomous and, hence, it may be easier to analyze these kinds of instabilities behaviours from a dynamical systems perspective, at least in principle.
1 The panel in the bottom-left also uses a smaller initial domain size from the others; see [4] for details.
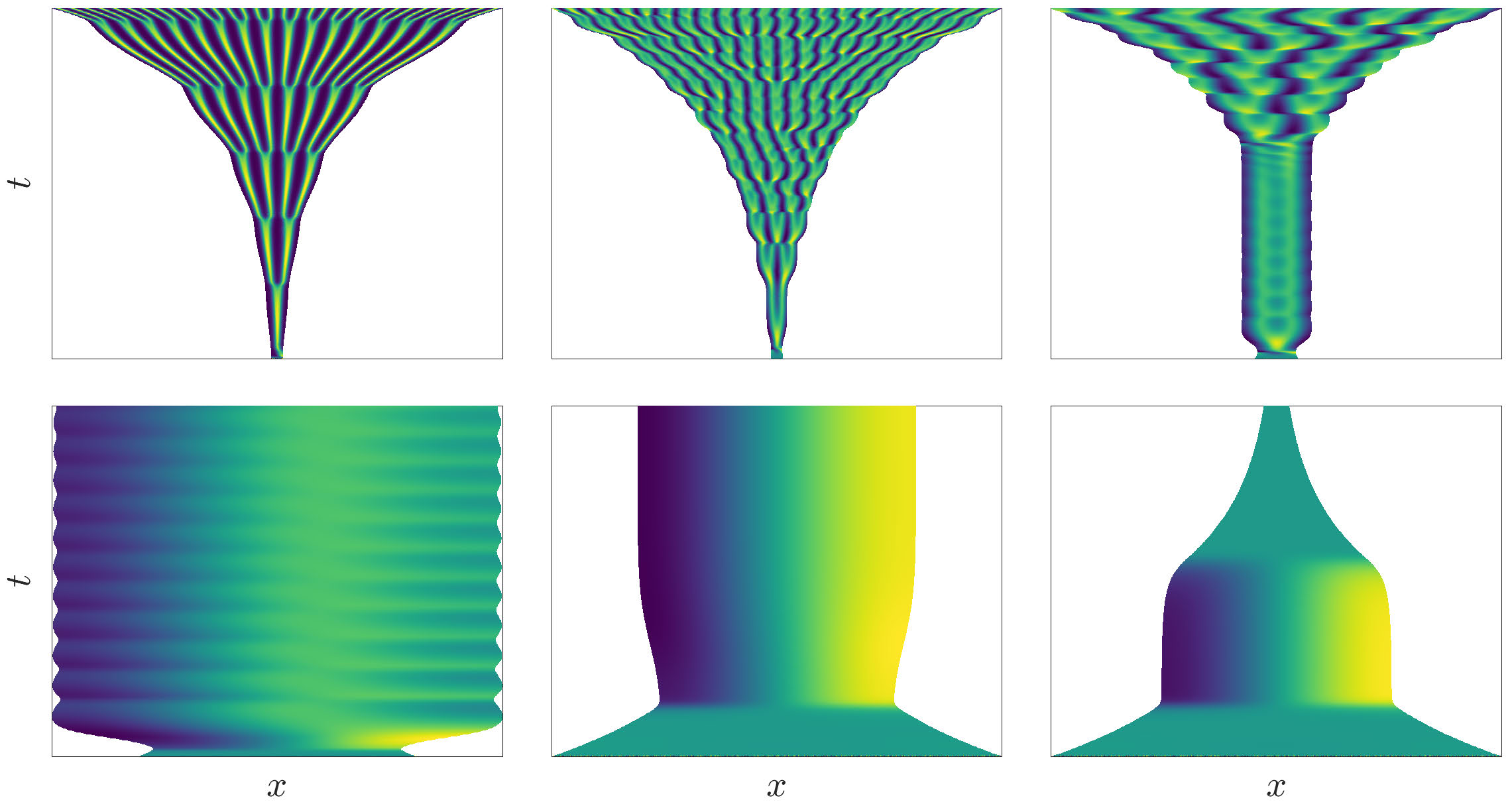
Figure 2: Simulations from a Schnakenberg reaction-diffusion simulation on a concentration-dependent domain that is locally contraction in regions of low concentration (dark blue) and growing in regions of high concentration (bright yellow).
Lastly, we mention an important aspect of higher-dimensional reaction-diffusion systems, relevant in all but the 1D case, and highlight the complexity of concentration-dependent dynamics. Simply put, developing constitutive theory for local-growth models is difficult, with no obvious link between local, concentration-dependent growth laws and the heavily studied coordinate-dilational or uniformly evolving manifolds. In [4], we posited the evolution of the volume form of the domain through a simple ODE, but further information is required to specify the evolution of the domain, a fact that does not apply to 1D problems. Even in our simple case, where we supplement this growth law with a further, mathematically convenient constitutive assumption, this was sufficient to give rise to rich and exotic dynamics, of breadth greater than the 1D examples shown above. Fig. 3 shows a simple example of this, illustrating the evolution of a single spot in a Gierer-Meinhardt model where the domain is only growing in regions of high concentration (i.e. where the spot is). The domain spontaneously extrudes in the direction of the spot, which then begins spiralling around, presumably following local gradients in curvature [3], significantly modifying the domain during the course of its evolution.

Figure 3: A simulation of concentration-dependent growth in a 2D domain. The left panel is the evolution of the domain boundary over time, and the right the evolution of the corresponding concentration profile.
These preliminary results indicate a plethora of possibilities in studying and designing reaction-diffusion systems with a range of different dynamical behaviours. As Turing himself said, "We can only see a short distance ahead, but we can see plenty there that needs to be done."
References
[1] E. J. Crampin, E. A. Gaffney, and P. K. Maini, Reaction and diffusion on growing domains: scenarios for robust pattern formation, Bulletin of Mathematical Biology, 61 (1999), pp. 1093-1120.
[2] E. J. Crampin, W. W. Hackborn, and P. K. Maini, Pattern formation in reaction-diffusion models with nonuniform domain growth, Bulletin of Mathematical Biology, 64 (2002), pp. 747-769.
[3] D. Iron and M. J. Ward, The dynamics of boundary spikes for a nonlocal reaction-diffusion model, European Journal of Applied Mathematics, 11 (2000), pp. 491-514.
[4] A. L. Krause, E. A. Gaffney, and B. J. Walker, Concentration-dependent domain evolution in reaction-diffusion systems, arXiv preprint, https://arxiv.org/abs/2209.02085.
[5] A. M. Turing, The chemical basis of morphogenesis, Philosophical Transactions of the Royal Society of London. Series B, Biological Sciences, 237 (1952), pp. 37-72.
[6] K.-I. Ueda and Y. Nishiura, A mathematical mechanism for instabilities in stripe formation on growing domains, Physica D: Nonlinear Phenomena, 241 (2012), pp. 37-59.
[7] R. A. Van Gorder, V. Klika, and A. L. Krause, Turing conditions for pattern forming systems on evolving manifolds, Journal of Mathematical Biology, 82 (2021).
| Author Institutional Affiliation | Andrew Krause (Durham University) and Benjamin Walker (University College London) |
| Author Email | |
| Keywords | pattern formation, patterns and simulations, evolving domains |