Design of parametrically forced patterns and quasipatterns
Transition from 12-fold to 14-fold quasipatterns
Quasipatterns are two-dimensional patterns that have clear rotational symmetry
on average, usually 8-, 10- or 12-fold; this symmetry is readily apparent in
their Fourier spectra. However, the plane cannot be covered periodically with
tiles having these rotational symmetries, and so quasipatterns do not have any
translational symmetry.
Quasipatterns were discovered in the Faraday wave experiment (in which a tray
of fluid is shaken up and down hard enough to form standing waves on its
surface), and are the fluid-mechanical analogue of quasicrystals - the
discovery of which earned Dan Shechtman the NobelPrize in Chemistry 2011.
In the Faraday wave experiment, 8, 10 and 12-fold quasipatterns have been
found, though Zhang and Vinals (1996)
anticipated that with a dimensionless surface tension equal to one third and in
the low viscosity limit, higher-order quasipatterns might also be found. In
these circumstances, plane waves in two directions hardly influence each other
at all, provided the directions are more that about 30 degrees apart.
In a partial differential equation with periodic forcing, developed in
doi:10.1137/080719066 as a qualitative model of the Faraday wave experiment, 12-fold quasipatterns are
found close to the onset of pattern formation (1.1 times critical), but on
driving the system harder (1.3 times critical), the 12-fold quasipatterns are
now unstable and are replaced by 14-fold quasipatterns, or by 20-fold
quasipatterns when the domain size is enlarged. These are the first examples
of quasipatterns of order greater than 12 found as stable solutions of a
partial differential equation.
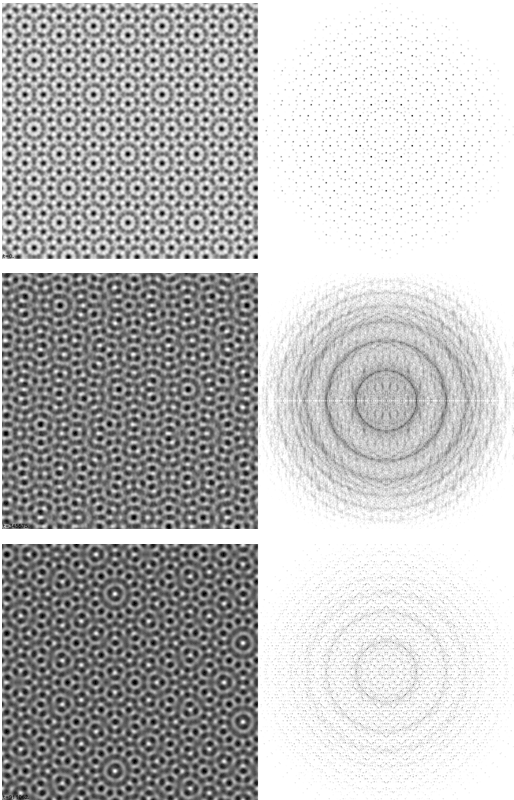
The three frames on the left illustrate the stages in the transition from 12-fold to
14-fold quasipattern, starting at 12-fold on the top, going through a state of
spatiotemporal chaos in the centre, before settling down to a 14-fold
quasipattern on the bottom. In each image, the left half is the pattern and the
right half is its Fourier transform.
The frame below on the left shows the time evolution of the amplitudes of the most
important Fourier modes, with those belonging to the 12-fold quasipattern in
red, and those belonging to the 14-fold quasipattern in green.
The movie of the transition is shown below via YouTube:
| Author Institutional Affiliation | Department of Applied Mathematics, University of Leeds, UK |
| Author Email | |
| Author Postal Mail | Department of Applied Mathematics / University of Leeds / Leeds LS2 9JT, UK / Phone: +44 113 343 5161 |
| Notes | published in SIADS 8(1): 298-347, 2009 |
| Keywords | Pattern formation, quasipatterns, superlattice patterns, mode interactions, Faraday waves |