The results in this article summarize those in W.D. Martinson, R. McLennan, J.M. Teddy, M.C. McKinney, L.A. Davidson, R.E. Baker, H.M. Byrne, P.M. Kulesa, and P.K. Maini, "Dynamic fibronectin assembly and remodeling by leader neural crest cells prevents jamming in collective cell migration", eLife 12 (2023): e83792 [3].

The coordinated movement of cells is critically important for developing embryos, as it supports the creation of new tissues that are essential for a healthy organism. Collective cell migration is exemplified by the neural crest, a stem cell population that emerges from a spinal cord precursor known as the neural tube. Largely immobile early in development, the neural crest eventually transitions to a migratory phenotype and, depending on where its cells ultimately travel, differentiates into various descendants including melanocytes (skin cells), osteocytes (bone cells), or even peripheral neurons. Unsurprisingly, disruptions to neural crest cell migration are associated with developmental disorders called neurocristopathies, such as Hirschsprung disease or Meckel-Gruber syndrome, that affect nerve formation, the skeletal system, and skin pigmentation [8].

Greater understanding of how neural crest cells control their movement towards target sites will thus inform new strategies to mitigate, or even prevent, the onset of these disorders. Answering this biological problem will also bring insight into an interesting mathematical question concerning the mechanisms underlying pattern formation, since groups of neural crest cells form anisotropic ribbon-like streams in the head that are robust to many experimental perturbations. Mathematical modeling of the neural crest [1, 7, 5, 6, 4] has been used to understand how repulsive interactions and cell-induced gradients of chemoattractants contribute to such patterning. It remains largely unclear, however, how the environments in which cells travel also affect the control, formation, and direction of neural crest streams. Furthermore, it is known that in silico streams created by cell-induced gradient models are highly susceptible to separation between leading and trailing cells that is not observed in vivo, suggesting a gap in the theoretical understanding of neural crest cell migration [2].

Our interdisciplinary group sought to answer these open questions by developing a new mathematical framework to capture the dynamic interaction between neural crest cells and their local microenvironment. The equations underlying our model are inspired by previous approaches and in vivo observations of fibronectin, a constituent protein of the extracellular matrix (ECM), during chick neural crest cell migration in the head. These investigations indicated that the fibronectin distribution prior to neural crest colonization exhibited a highly discrete and punctate structure. By contrast, after neural crest cells had migrated the distribution appeared to contain fibrous scaffolds similar to those observed in mature organisms. This led us to hypothesize that neural crest cells secrete and construct fibrous ECM scaffolds during the course of their migration that supports the creation of stream patterns that are robust to separation between leading and trailing cells.

Our mathematical model, which tracks each individual cell, assesses the plausibility of this hypothesis. It consists of a system of differential equations based on an overdamped version of Newton's second law that accounts for frictional forces, cell-ECM forces, and cell-cell repulsive forces:

\( \frac{\text{d}\mathbf{x}^{(i)}}{\text{d}t} = \mathbf{v}^{(i)}_\text{ECM} + \mathbf{v}^{(i)}_\text{rep}, \)

(1)

where \(\mathbf{x}^{(i)}\) denotes the \(i^\text{th}\) cell's position in a 2D plane. The ECM is represented as discrete points in the plane, however to model the formation of a fibrous scaffold we associate an angle to each point that has been passed over by a cell, such that a vector field representing the ECM scaffold is created over the course of a simulation. The magnitudes of cell-cell and cell-ECM forces are computed as though individuals are attached to neighboring cells or ECM molecules with nonlinear springs. The direction of the cell-cell force is sampled randomly from distributions biased towards lower cell numbers. The direction of the cell-ECM force is sampled in a similar fashion, but in this case the distribution is biased so that cells move towards greater numbers of ECM molecules (haptotaxis), align themselves along the vectors it senses within a local neighborhood (contact guidance), or a linear combination of the two.

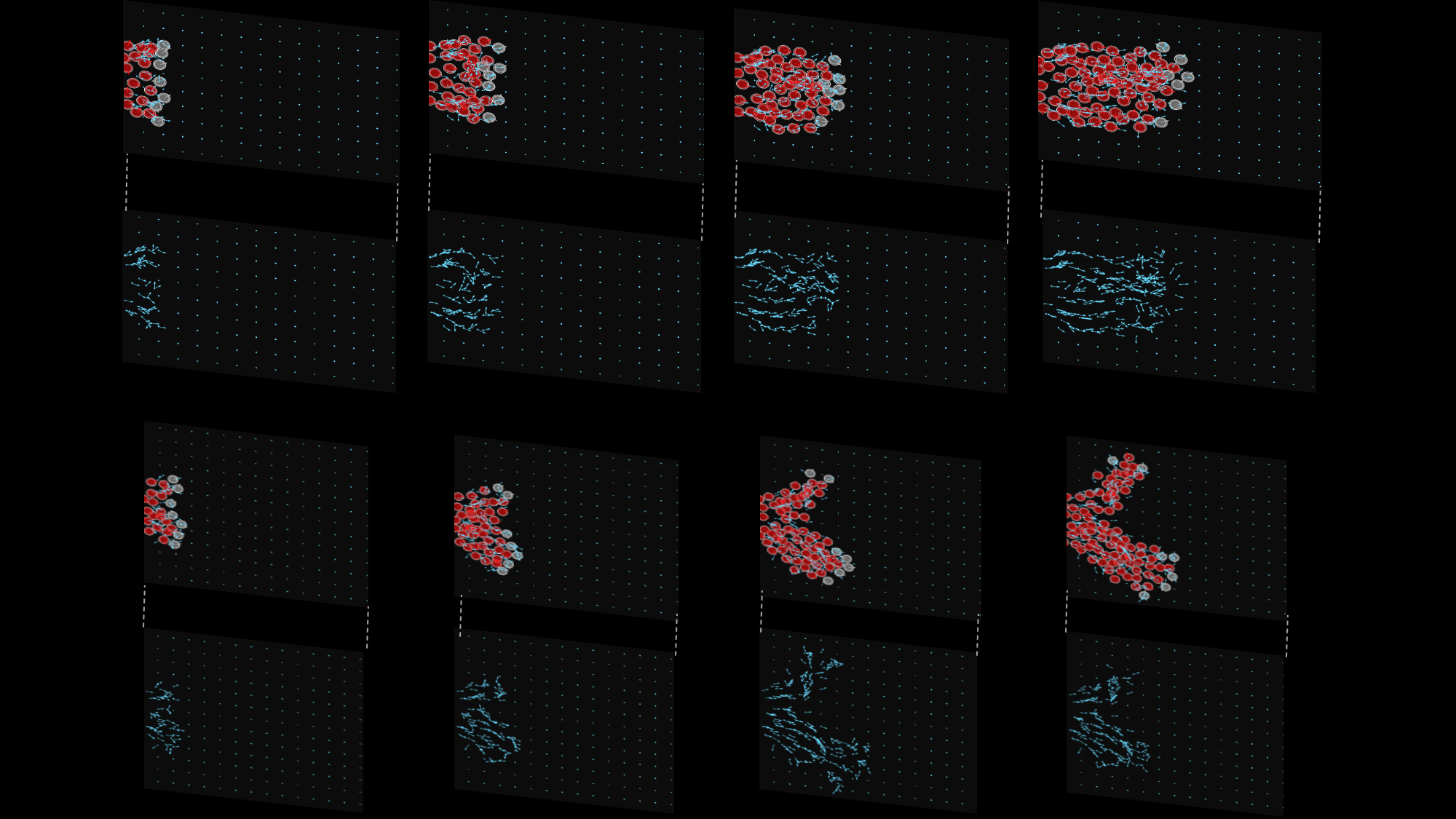
Figure 1: This image depicts results from two example ABM simulations (first and third rows: cell and ECM plotted together, second and fourth rows: only ECM). Fibronectin puncta and fibers are denoted by light blue squares and arrows, respectively, while neural crest cells are depicted as red and grey circles. Grey neural crest cells are initialized at the head of the stream and can create ECM molecules in the domain, while red neural crest cells enter the domain over the course of the simulation from the left boundary and can only remodel existing ECM molecules. Snapshots across the four columns are taken in intervals of 180 simulated minutes. The simulations use the same parameter values but different random seeds. They demonstrate that streams of cells tend to form over the course of the simulation. However, cells are unable to robustly follow the correct target corridor (horizontal axis) unless an additional mechanism to guide leading cells is included. Adapted from [3] and covered by a CC-BY 4.0 DEED license (https://creativecommons.org/licenses/by/4.0/).

Our preliminary investigations of the mathematical model highlighted how cell remodeling of the ECM was important in establishing long-distance anisotropic streams (Fig. 1 and Movies 1-4). The distance that cells traveled was most sensitive to the degree by which cells align themselves along ECM fibers. We confirmed these observations by performing a global sensitivity analysis of the model in which multiple parameters were perturbed simultaneously. Simulated knockdown and gain-of-function experiments further indicated that cells at the front of streams were most critical for controlling the eventual direction in which the collective travels. By adding a third force in the model that was always directed along the target corridor, which could represent mechanisms like chemotaxis that are not directly incorporated into the modeling framework, we generated stream breaks between leading and trailing cells. Simulated experiments that rescued the single stream pattern suggested that contact guidance is a key mechanism for controlling the robustness of streams (i.e., trailing cells can more efficiently sense and travel to leaders by aligning more closely along the ECM scaffold).

This work is useful because it highlights how the reciprocal interactions between cell movement and ECM remodeling can play a significant role in supporting collective cell migration. By establishing and continually reinforcing a scaffold, leading cells can efficiently and accurately communicate their migratory paths to trailing cells, even over large spatial and/or temporal scales. Furthermore, we observed that upregulating mechanisms related to ECM remodeling allowed cells to overcome so-called "jammed" states associated with high cell density and little to no migration. Our theoretical model provides verifiable predictions that is currently guiding the design of new experiments, and thus serves as an example of how mathematics can bring insight into biology, and vice versa.

Movie 1: A movie depicting the top row of Fig. 1, in which a single stream of neural crest cells (red and grey circles) forms. Cells interact with, and influence the dynamics of, ECM molecules and fibers (blue points and arrows). Adapted from [3] and covered by a CC-BY 4.0 DEED license (https://creativecommons.org/licenses/by/4.0/).

Movie 2: This movie depicts only the ECM molecules and fibers associated with Movie 1. It is associated with the second row of Fig. 1. Adapted from [3] and covered by a CC-BY 4.0 DEED license (https://creativecommons.org/licenses/by/4.0/).

Movie 3: A movie depicting the stochastic simulation also shown in the third row of Fig. 1, in which a single stream of neural crest cells (red and grey circles) forms and eventually splits into two moving branches. This stochastic simulation uses the same parameter values as in Movies 1-2. While cells tend to maintain a coherent group (as in Movie 1) across random seeds, additional mechanisms are necessary to ensure no stochastic simulations lead to bifurcating streams. Adapted from [3] and covered by a CC-BY 4.0 DEED license (https://creativecommons.org/licenses/by/4.0/).

Movie 4: This movie depicts only the ECM molecules and fibers associated with Movie 3. It is associated with the fourth row of Fig. 1. Adapted from [3] and covered by a CC-BY 4.0 DEED license (https://creativecommons.org/licenses/by/4.0/).

References:

[1] A. Colombi, M. Scianna, K. J. Painter, and L. Preziosi, Modelling chase-and-run migration in heterogeneous populations, J. Math. Biol., 80 (2020), pp. 423-456.

[2] R. Giniunaite, R. McLennan, M. C. McKinney, R. E. Baker, P. M. Kulesa, and P. K. Maini, An interdisciplinary approach to investigate collective cell migration in neural crest, Dev. Dyn., 2019, pp. 1-11.

[3] W. D. Martinson, R. McLennan, J. M. Teddy, M. C. McKinney, L. A. Davidson, R. E. Baker, H. M. Byrne, P. M. Kulesa, and P. K. Maini, Dynamic fibronectin assembly and remodeling by leader neural crest cells prevents jamming in collective cell migration, eLife, 12 (2023).

[4] M. C. McKinney, R. McLennan, R. Giniunaite, R. E. Baker, P. K. Maini, H. G. Othmer, and P. M. Kulesa, Visualizing mesoderm and neural crest cell dynamics during chick head morphogenesis, Dev. Biol., 461 (2020), pp. 184-196.

[5] R. McLennan, L. Dyson, K. W. Prather, J. A. Morrison, R. E. Baker, P. K. Maini, and P. M. Kulesa, Multiscale mechanisms of cell migration during development: Theory and experiment, Development, 139 (2012), pp. 2935-2944.

[6] L. J. Schumacher, Neural crest migration with continuous cell states, J. Theor. Biol., 481 (2019), pp. 84-90.

[7] A. Szabó, M. Melchionda, G. Nastasi, M. L. Woods, S. Campo, R. Perris, and R. Mayor, In vivo confinement promotes collective migration of neural crest cells, J. Cell Biol., 213 (2016), pp. 543-555.

[8] G. A. Vega-Lopez, S. Cerrizuela, C. Tribulo, and M. J. Aybar, Neurocristopathies: New insights 150 years after the neural crest discovery, Dev. Biol., 444 (2018), pp. S110-S143.