My name is Andrea Welsh and I am is a new postdoctoral researcher working with Prof. Bard Ermentrout at University of Pittsburgh. I received my Ph.D. in Physics from Georgia Institute of Technology Fall 2019 for my dissertation called “Coming together: Individuals at Different Scales working For a Common Goal” under the advisement of Prof. Flavio Fenton. I received my Bachelor of Arts from Boston University in 2011 in physics and math. I am interested in projects involved with pattern formation and dynamical systems and I am also interested in equity and inclusion, with a personal focus on LGBTQIA+ and mental health advocacy. 

Touching on D&D Probabilities: 

One thing I do in my free time is play a tabletop roll playing game (TTRPG) called Dungeons and Dragons (D&D). I am going to discuss just a few fun mathematical topics that come up while playing this game.

For those of you who have not had the pleasure of playing, this is a game played with a group of people and involves both a creative element and also math. One person is called the Dungeon Master (DM) who creates the story, world building, and challenges for the rest of the players who act as characters that they have created. These characters have certain abilities and actions that they can take, the success of with depends on dice rolls. Each D&D player has a set of polyhedral dice to roll in different situations, but the main die is the icosahedron which is often just called the D20. For many checks, player rolls the D20 and may add points depending on what ability they are checking and what sort of personal skills their character has, and this is compared against either a value predetermined by the DM or another die roll. Additionally, rolling a 20 typically is called a critical success and often gives a more positive outcome (rolled damage gets doubled) and rolling a 1 is called a critical failure and may lead to negative consequence depending on house rules and the DM. For other sorts of actions, such as physical attacks or spells, polyhedral dice with 4, 6, 8, 10 or 12 may be used. 

A player also has different stats that can be determined by dice rolls, point buy, or a stat block for the six ability scores called strength, constitution, dexterity, intelligence, wisdom, and charisma. I won’t get into the specifics the different ways to determine these numbers or what they mean, but these scores are whole positive or negative numbers typically between -3 to +3 that get added to skill checks, attacks, and determine properties about your character.

One example is your “armor class” (AC), or the minimum number that someone else would need to roll in order to successfully attack your character by making an “attack roll,” which is calculated by adding 10 to your dexterity score and whatever bonuses you get from different equipment. These values are also is used in calculating your character’s spell “difficulty class” (DC), or the minimum number that a defending player needs to roll in order to resist the effects of a spell when they make a “saving throw”. There is some quick mathematics that can be used here to figure out whether or not it is better to make an attack roll to beat the defending person’s AC or use a spell where the defending person needs to make a saving throw to beat your DC. This involves trying to figure out not only the probabilities of rolling the D20 and getting above the AC or below your DC, but also some estimated guesses on what sort of values the person may have for their ability scores that they will then add to their rolls (casting the spell “Bane” which requires a charisma saving throw of 15 for my level 8 Druid may be a better choice against an Animated Armor with a charisma score of -5 than during an attack roll against their AC of 18 because then the Animated Armor can only roll between -4 and 15 yielding only a 5% chance of success against my DC of 15 vs my spell attack bonus of +7 making my rolls between 8 and 27 yielding a 60% change of success against their AC of 18). But now we are getting a little bit in the weeds on the field…

Prepare for battle: The L-infinity norm

One part of the game is exploration. The D&D may set the scene of where the player characters are by describing what they see and where everything is. If it is important for the players to see what is around there may be a map which is drawn on grid of 1x1 inch squares which correspond to 5x5ft.

There are instances, such as during battle, where the number of squares someone can move is limited during a turn. Each character has a speed which is given feet (because time units are set to equal 1) that is a multiple of 5 and can move in any of the 8, and so a metric needs to be set. There is the standard metric has every square equal five feet of movement regardless of the direction the character moves. However, we can see that when moving in a diagonal way, that if we make all directions equal distance, we will get some odd measurements. The figure shows that this typical movement metric leads our characters moving in a L-infinity norm. In this norm, spells which have a spherical or cylindrical area of affect, actually take up a large square on the map, even if the space is large enough that a circle can be measured out on the map that will remove some squares from the space. Call Lightning which affects a 10 foot tall, 60-foot radius cylinder is actually taking up a 10 x120x120 rectangular prism which is much larger!

However, some DMs chose to use the taxicab distance which is the \(L_1\) norm that we are used to. Each square becomes 5ft of movement or space no matter what direction. In this case, players wouldn’t be able to move diagonally and have to effectively move as if they are driving blocks in Manhattan in order to get from point A to point B. In either case, we are dealing with a space that is non-Euclidean. Finally, the DM’s Guide has a special case of diagonal movement where the first diagonal uses 5ft of movement, the second 10ft, the next 5ft, the following 10ft again and so on. This ends up being more accurate when it comes to physical space on the board and the distance a player can travel or the area a spell takes up. Unfortunately, as players increase in level and gain items to cause their speeds to increase, even this metric becomes more and more inaccurate. It also doesn’t help that during the heat of a battle when actions need to happen pretty quickly, it is the more complicated of the 3 metrics to count out, leading to stress and likely errors.

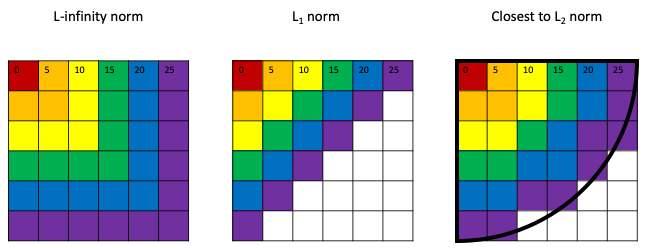
Perhaps in the future other metrics will become more commonly used as mathematicians continue to think of ways to make the game more realistic. There may not be a perfect way of measuring distances in this game, but part of the fun is arguing with the DM and coming together with other players to help puzzle out just the best way your Water Genasi eldritch knight can help stop the beholder in time. There are lots of other ways I get to use math and physics to help me out during this game (such as: “are your dice really not symmetric or are you just very unlucky?” and “if I roll enough 1s, I am due for a natural 20 any time now” ), but I hope this at least gets you interested enough to get a group of cohorts together and try it out.